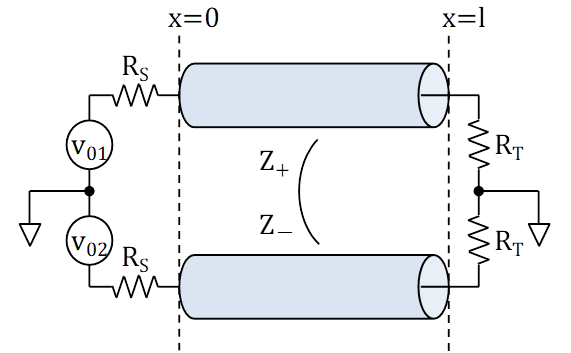
Multiple reflection in terminated coupled-lines
|
Two coupled-lines are identically terminated.
The voltage and current at the source end are:
The voltage and current at the termination end are:
where τ is the propagation delay and ΓS,ΓT are the reflection coefficients.
|
We will derive a special solution for identically terminated coupled-lines.
***
We assume the boundary condition at the source and termination end as:
![]()
![]()
Because the terminations of two lines are identical, it is convenient to use even and odd mode.
![]()
![]()
Laplace transformed boundary conditions are:
![]()
![]()
Reminding that these conditions and the general solutions (→General solution of coupled transmission-line equations)
![]()
![]()
are the similar form in the case of a single line (→Multiple reflection in a terminated line) ,we obtain the solutions:
![]()
![]()
where τ is the propagation time from x=0 to x=l and ΓS,ΓT are the reflection coefficients.
![]()
***
The voltages of line-1 and 2 are obtained from those of even and odd mode:
![]()