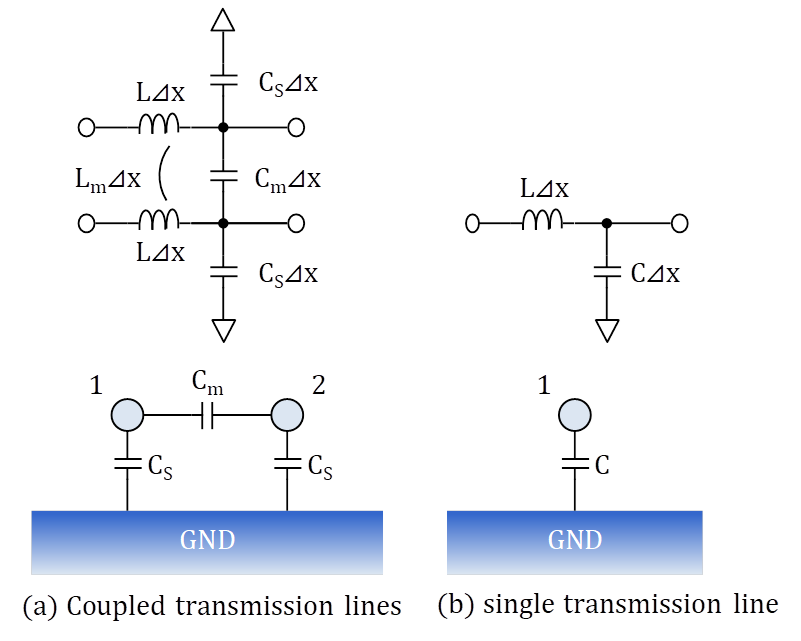
Differential and single-ended impedances
|
Assuming that the propagation speed of TEM wave is constant:
the even and odd mode impedances are:
where Z0 is the characteristic impedance of each line:
and ξ is a parameter that indicates the strength of coupling:
Conversely,
|
We will derive the relation between differential and single-ended impedances.
***
First, we will derive the relation among capacitances Cs, Cm and C.
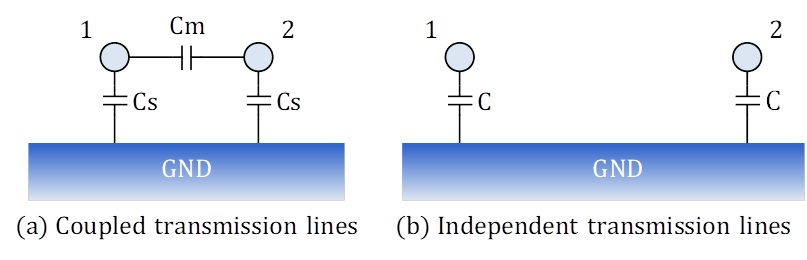
Assuming the line-1 exists alone with line-charge q1, its voltage is:
![]()
Superposing the electric field of the line-2 charged with q2, the line-1 voltage can be expressed with a parameter ξ:
![]()
Because the line-1 and line-2 are identical, the line-2 voltage is similarly expressed:
![]()
![]()
If the line-1 and line-2 voltages are held at v1 and v2, the line-charges are:
![]()
Comparing the coefficients of two equations, we obtain the relation among Cs, Cm and C:
![]()
![]()
Noting that Cs≦C and 0<Cm,C because of physical implications, then
![]()
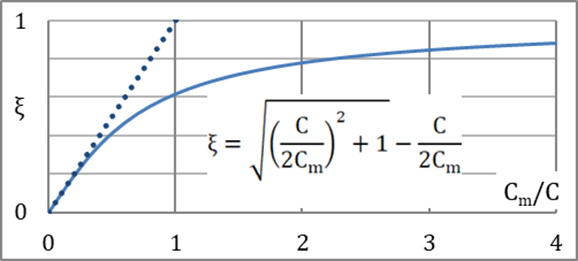
Solving eq. (2) for the positive ξ:
![]()
This parameter ξ increases according to Cm/C, so it indicates the strength of coupling.
***
In the previous section, we have expressed Z± and u± in terms of L,Lm,Cs,Cm:
![]()
![]()
Inserting (1) and (2) into these equations, we obtain the expression of Z± and u± in terms of L,Lm,ξ:
![]()
![]()
where Z0 and u is the characteristic impedance and propagation speed of each line:
![]()
Although u+ and u− look different from each other, the propagation speed of TEM wave must be a constant, that is the light speed. So assuming
![]()
, the inductances are also expressed by the parameter ξ:
![]()
Then we can simply express the even and odd mode impedances in terms of Z0 and ξ :
![]()
Noting 0≦ξ<1 because of physical implications, then
![]()
Conversely, Z0 is the arithmetic average of Z+ and Z-, and ξ is the relative difference between Z+ and Z-:
![]()