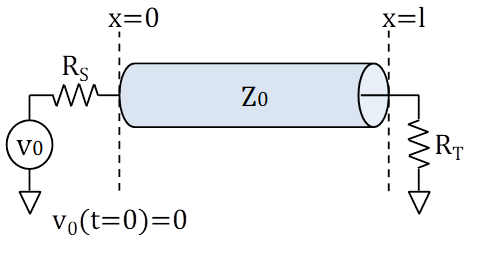
Multiple reflection in a terminated line

<prev | up | next>

---

A transmission line is terminated by R_S and R_T at the source and termination end respectively.

The voltage and current at the source end are

The voltage and current at the termination end are

where τ is the propagation delay and Γ_S,Γ_T are the reflection coefficients.

Assuming the input voltage v₀(t) is a rising step.

The voltage and current at the source end are

The voltage and current at the termination end are

We will derive a special solution for a terminated line.

***

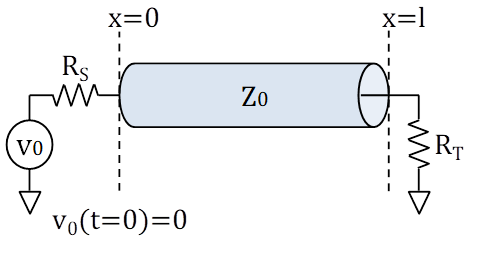
We assume the boundary conditions at the source and termination end as

Laplace transformed boundary conditions are

Inserting general solutions

into the boundary conditions, we obtain the equations in terms of V_a and V_b

Combining like terms:

Using the propagation delay τ and reflection coefficients Γ_S,Γ_T instead of l and R_S,R_T,

then

Eliminating V_b, we obtain V_a

Here we expand 1/(1-x) to move the factor exp(-2τs) from the devisor to dividend. This geometric series represents the multiple reflection.

Substituting the V_a and V_b into the general solutions, we obtain the special solutions in the 's' domain

Noting that multiplying exp(-τs) in the 's' domain is equivalent to shifting by τ(>0) in the time domain

,then we obtain the special solutions in the time domain.

***

The voltage and current at the source end (x=0) are

These solutions satisfy the boundary condition.

***

By noting l/u=τ, the voltage and current at the termination end (x=l) are

These solutions satisfy the boundary condition.

***

We assume that the input voltage v₀(t) is a rising step.

Using the identity:

, the voltages can be expressed in terms of the amplitude in nτ< t <mτ: v₀(t-nτ)-v₀(t-mτ)

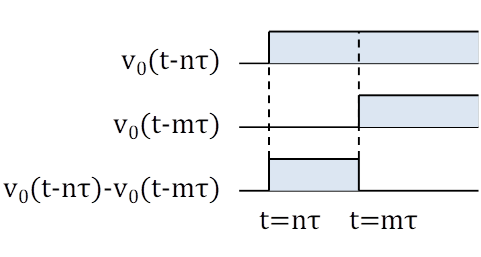
At the source end:

At the terminaton end:

or, explicitly

At the source end:

At the terminaton end:

Moreover expressing the amplitude in terms of R_S,R_T and Z₀,

At the source end:

At the terminaton end:

***

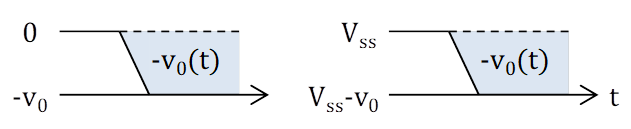
So far we have assumed the initial condition as v(x,0)=0,i(x,0)=0. When the source voltage V_SS or termination voltage V_TT is not zero,

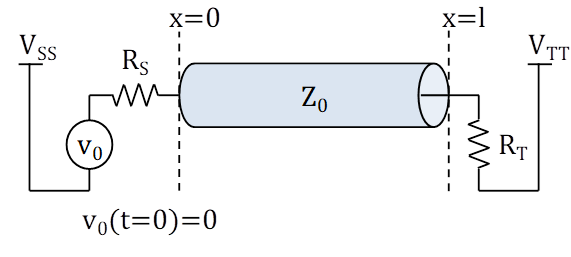
we may just superpose the DC circuit.

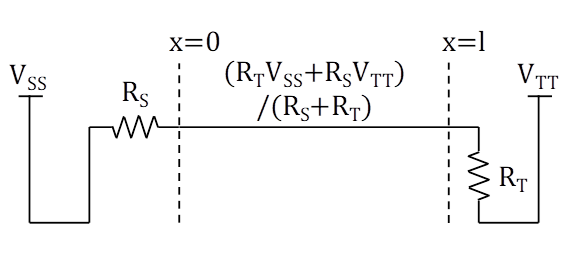
Then the solution is just shifted by constant.

For example, a transition from HIGH(V_SS) to LOW(0V) is equivalent to a transition from 0V to negative voltage.

---

<prev | up | next>