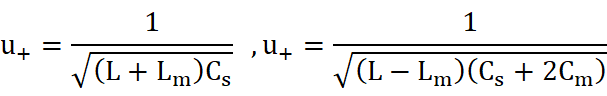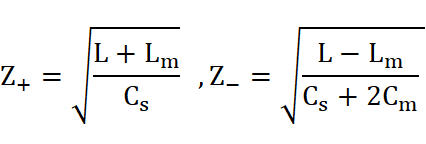General solution of coupled transmission-line equations

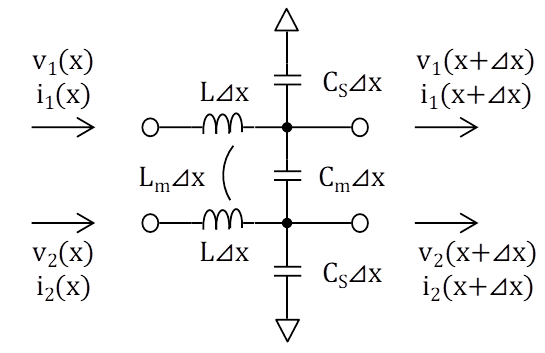
Equations of coupled transmission lines:

The voltage and current in even and odd mode:

Initial conditions:

General solutions in Laplace transform 's' domain:

General solutions in the time domain:

where u_± are the propagation speed and Z_± are the characteristic impedance:

We will derive the general solution of the coupled transmission-line equations.

***

Equations of coupled transmission lines are:

Using the even and odd mode instead of each line variables

, the equations will be diagonalized:

Eliminating the i_±, we obtain the wave equation of v_±:

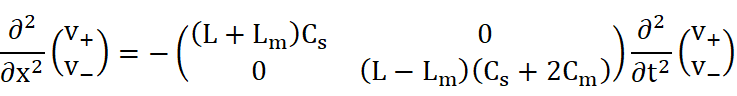
As in the case of a single transmission line (→General solution of transmission-line equations), assuming the initial condition

, the general solutions in Laplace's transform 's' domain are:

and the solutions in the time domain are:

where u_± are the propagation speeds and Z_± are the characteristic impedances in even and odd mode:

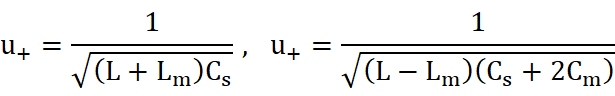
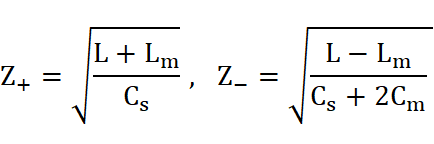
The expressions of u₊ and u_－ look different, but they must be equal in the case of TEM wave. We will confirm it later by an example.(→Differential impedance of two wires over a ground plane)