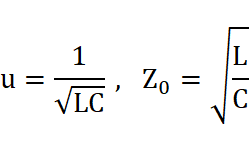General solution of transmission-line equations
|
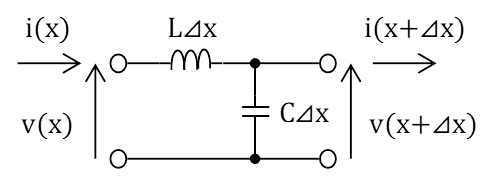
Equations of a transmission line:
Initial conditions:
General solutions in the Laplace transform 's' domain:
General solutions in the time domain:
where u is the propagation speed and Z0 is the characteristic impedance:
|
We will derive the general solution of transmission line equations.
*
Equations of a transmission line are:
![]()
The Laplace transform of the voltage v(x,t) and current i(x,t) are:
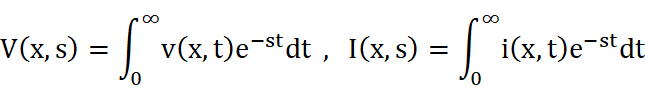
and the Laplace transform of its time derivative is:
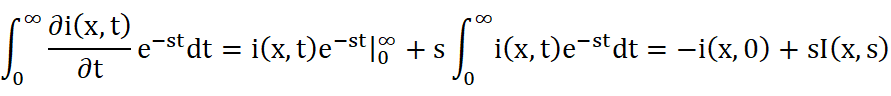
![]()
Therefore, the Laplace transform of the equations are:
![]()
![]()
Here we assume the initial conditions at t=0 as:
![]()
, then we obtain the wave equation:
![]()
Its general solutions are:
![]()
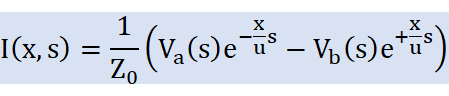
Using the propagation speed u and the characteristic impedance Z0 instead of L and C:
![]()
![]()
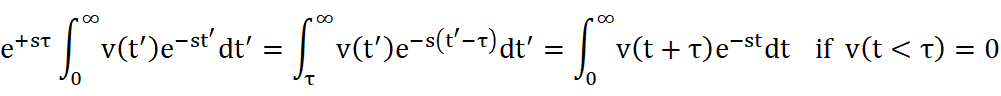
**
Multiplying exp(-Ńs) in the 's' domain is equivalent to shifting in the time domain by Ń.
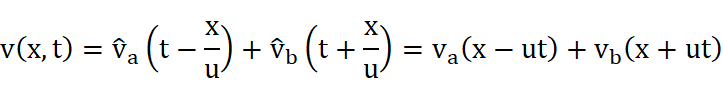


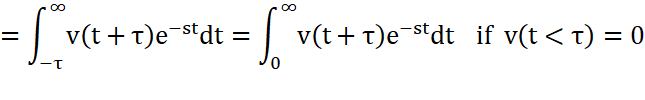
Therefore the general solutions in the time domain are:
![]()

where the va/vb are forward/backward moving waves at the propagation speed u.