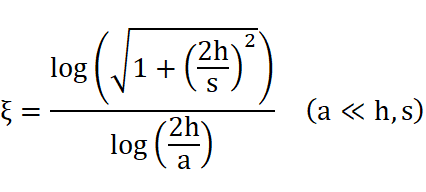Differential impedance of two wires over a ground plane
|
The even and odd mode impedances of two wires over a ground plane are:
where Z0 is the characteristic impedance of each line:
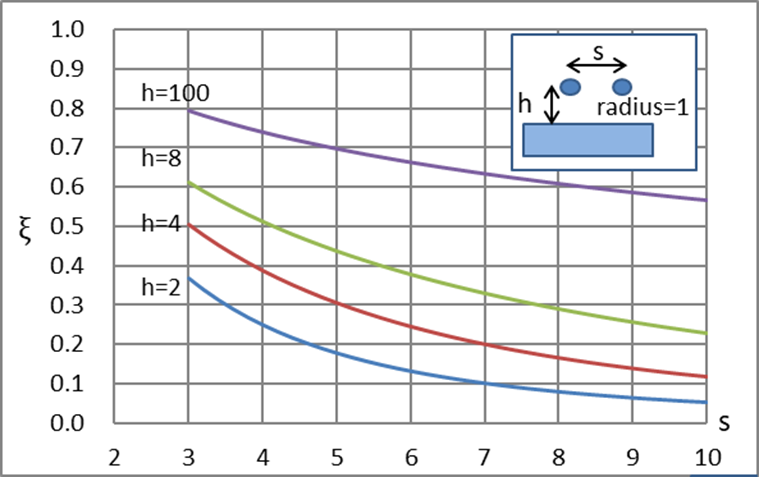
and ξ is a parameter that indicates the strength of coupling:
|
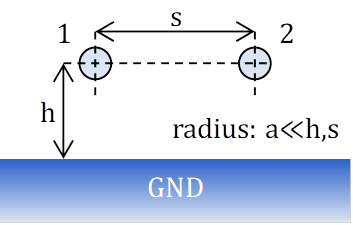
We will calculate the even and odd mode impedances, using an analytically solvable example: two wires over a ground plane. For convenience, we assume the radius of wire is negligibly small.
***
It is convenient to replace the ground plane with the mirror image of line-1 and 2.

***
First, we will calculate the self-capacitance of each line-1 and 2 and the mutual capacitance between line-1 and 2.
According to Gauss's law, the electric field E created by a line-charge q is:
![]()
Therefore, the potential at distance r from the line is:
![]()
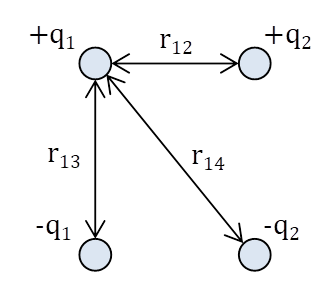
Let the potential at the ground plane be zero, the potential of the line-1 created by the charge of line-1,2,3 and 4 is:
![]()
![]()
Similarly, the potential of the line-2 created by the charge of line-1,2,3 and 4 is:
![]()
Expressing the line distances in terms of s and h,
![]()
we obtain the total potential of the line-1 and 2 created by line charges:
![]()
where F and Fm are the form factors:
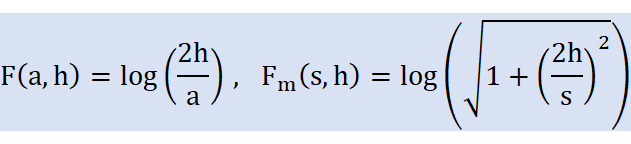
Conversely, the line-charges are:
![]()
The line-charges can also be expressed in terms of the capacitances Cs,Cm:
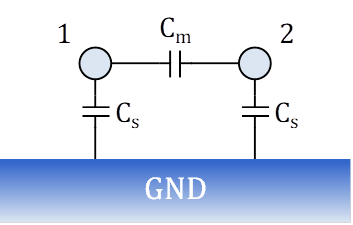
![]()
Comparing the coefficients of these equations, we obtain the self-capacitance of each line-1 and 2 and the mutual capacitance between line-1 and 2:
![]()
![]()
***
Next, we will calculate the self-inductance of each line-1 and 2 and the mutual-inductance between line-1 and 2.
According to Ampere's law, the magnetic field H created by a line-current I is:
![]()
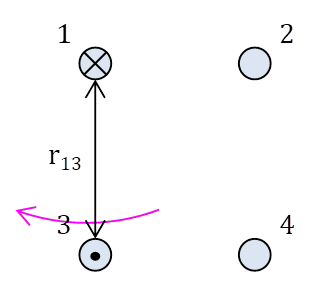
The magnetic flux created by the current of line-1, passing between line-1 and 3, is:
![]()
Therefore, the total magnetic flux created by the current of line-1 and 3 is:
![]()
The magnetic flux between line-1 and the plane is half of that between line-1 and 3. So we obtain the self inductance of line-1:
![]()
The mutual inductance between line-1 and 2 can be similarly calculated.
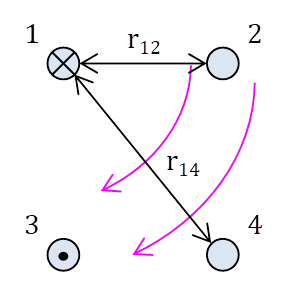
The magnetic flux created by the current of line-1, passing between line-2 and 4, is:
![]()
Then the total magnetic flux created by the current of line-1 and 3 is:
![]()
The magnetic flux between line-2 and the plane is half of that between line-2 and 4. So we obtain the mutual inductance between line-1 and 2:
![]()
Expressing the line distances in terms of s and h,
![]()
we obtain the self inductance and mutual inductance per unit length:
![]()
![]()
where F and Fm are the same form factors as those of the capacitances.
***
Using the capacitances and inductances above, we can calculate the propagation speeds in even and odd mode:
![]()
![]()
The form factors vanished, so we have confirmed that the propagation speed does not depend on the cross-section of conductors. Furthermore the propagation speeds in even and odd mode are just equal to the light speed in the media.
Similarly, the characteristic impedances in even and odd mode can be calculated:
![]()
![]()
Reminding that the characteristic impedance of a single wire over a ground plane is
![]()
,we can express the even and odd mode impedances in terms of Z0 and a parameter ξ:
![]()
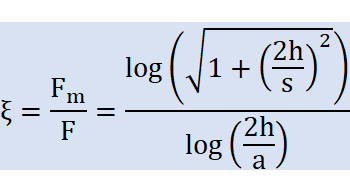
This parameter ξ increases as the line distance becomes closer.