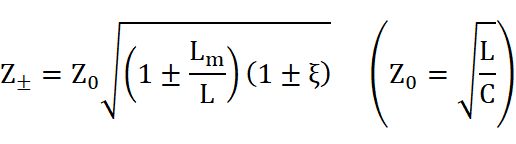Quasi-TEM approximation of impedance and propagation speed
|
In the quasi-TEM approximation, the even and odd mode impedances are:
and the propagation speeds are
where the parameter ξ is expressed in terms of capacitances:
|
So far, we have assumed that the propagation speeds in even and odd mode are equal.
![]()
This assumption does not hold if the electromagnetic wave is not in the TEM-mode. In the case of microstrip line, for example, the wave propagates with different speed in the substrate and air. The speed in even mode is always slower than that in odd mode, because the field in even mode mainly propagate in the substrate where the speed is slower than in the air. Then the deviation from a pure transversal wave increases as the wave propagates.
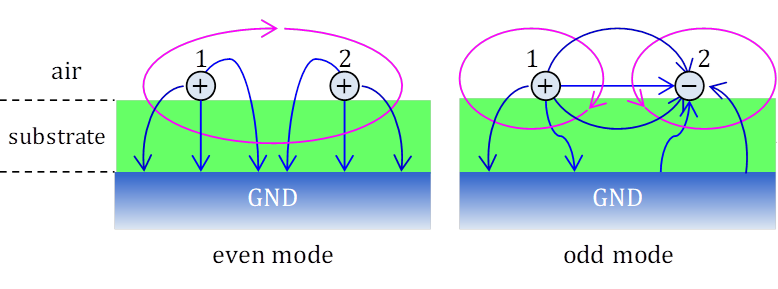
***
But, if the transmission line is not so long, the wave can be considered in the TEM mode approximately (quasi-TEM approximation). Using the distributed L and C model, we can calculate the characteristic impedance and propagation speed. (→Differential and single-ended impedances)
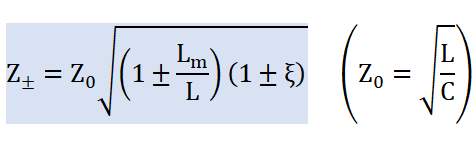
![]()
where ξ is a parameter expressed in terms of Cm/C:
![]()
Note that the following conditon is required for the pure TEM mode:
![]()
Some numerical values are computed below.
|
Cm/C |
ξ |
Lm/L |
u+/u |
u-/u |
Z+/Z0 |
Z-/Z0 |
|
|
0.2 |
0.193 |
0.3 |
0.96 |
1.07 |
1.25 |
0.75 |
quasi-TEM |
|
0.3 |
0.277 |
0.3 |
0.99 |
1.02 |
1.29 |
0.71 |
quasi-TEM |
|
0.330 |
0.3 |
0.3 |
1.00 |
1.00 |
1.30 |
0.70 |
TEM |
|
0.3 |
0.277 |
0.4 |
0.96 |
1.10 |
1.34 |
0.66 |
quasi-TEM |
|
0.4 |
0.351 |
0.4 |
0.98 |
1.04 |
1.38 |
0.62 |
quasi-TEM |
|
0.476 |
0.4 |
0.4 |
1.00 |
1.00 |
1.40 |
0.60 |
TEM |
|
0.5 |
0.414 |
0.5 |
0.97 |
1.08 |
1.46 |
0.54 |
quasi-TEM |
|
0.667 |
0.5 |
0.5 |
1.00 |
1.00 |
1.50 |
0.50 |
TEM |
|
0.2 |
0.193 |
0.3 |
0.96 |
1.07 |
1.25 |
0.75 |
quasi-TEM |