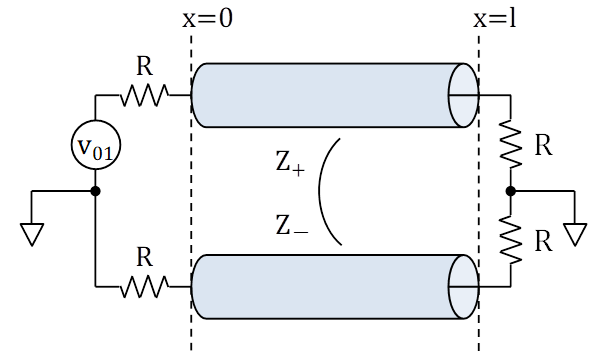
Crosstalk between two terminated lines (RS=RT=Zg)
|
Active line-1 and passive line-2 are terminated by a common resistance R at both ends.
The voltages at both ends of active line are:
The voltages at both ends of passive line are:
where
Especially if R is equal to the geometric average of Z+ and Z−:
then the voltages at both ends of active line are:
and the voltages at both ends of passive line are:
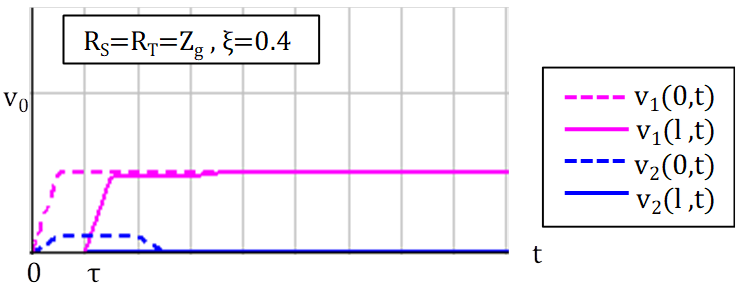
Therefore no crosstalk pulse induced at near end of active line and at far end of passive line. An example waveform for a rising step input v01(t) with rise time tr=τ/2:
Focusing on the first wave (n=0), the voltage on passive line is:
where ξ is the crosstalk coefficient:
|
We will calculate the crosstalk between two identical lines, terminated by a common resistance R at both ends.

***
The boundary conditions are:
(1) The termination resistances are equal at both ends : ΓS+=ΓT+=Γ+ , ΓS-=ΓT-=Γ-
(2) The propagation speeds are equal between even and odd mode : τ+=τ-=τ (TEM mode)
Then the voltages on line-1,2 results in more simple form.
Active line:
![]()
![]()
Passive line:
![]()
![]()
***
Here we assume the special condition that R is equal to the geometric average of Z+ and Z-:
![]()
This means that the reflection coefficients Γ+ and Γ- are equal in magnitude but opposite in sign:
![]()
Note that Γ+ can never be equal to Γ- because Z- is always larger than Z+ i.e. Γ+<Γ-.
Substituting Γ+=-Γ- into the equations above:
Active line:
![]()
![]()
Passive line:
![]()
![]()
Therefore no crosstalk pulse induced at near end of active line and at far end of passive line.
***
We will compute an waveform using these equations. For convenience, we express the reflection coefficient
![]()
with the crosstalk coefficient ξ and the normalized resistance r:
![]()
Reminding that the R is chosen to equal to the geometric average of Z+ and Z-:
![]()
Substituting this resistance, we obtain the reflection coefficients:
![]()

Assuming the input voltage v01(t) is a rising step with rise time tr=τ/2, and rS=1, ξ=0.4, the waveform is:
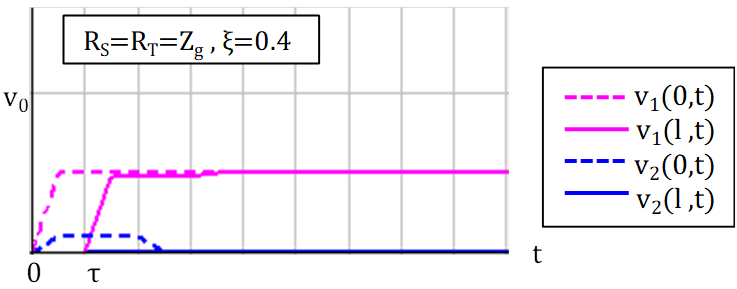
Note that no crosstalk pulse induced at near end of active line and at far end of passive line.
***
For more intuitive understanding, we will simplify the equation above. We assume that the input voltage v01(t) is a rising step.
![]()
Using the identity
![]()
, we obtain the voltages on line-1,2 in terms of the amplitude in nτ< t <mτ: v01(t-nτ)-v01(t-mτ),
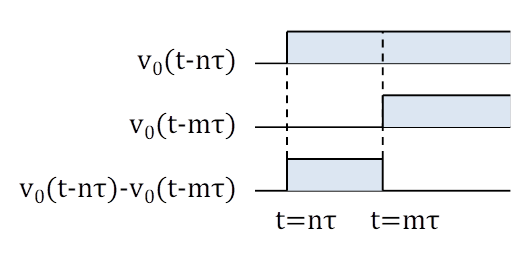
Active line:
![]()
![]()
Passive line:
![]()
![]()
or, explicitly
Active line:
![]()
![]()
Passive line:
![]()
![]()
***
Because of |Γ|<1, the amplitude attenuates exponentially with time. Then we will focus on the first wave (n=0) on passive line:
![]()
Because of 0≦ξ<1, its polarity is the same as the input voltage. And the amplitude is almost proportional to the crosstalk coefficient ξ.