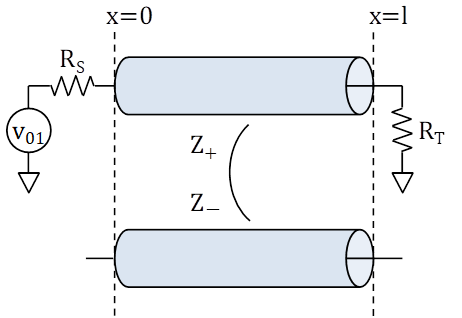
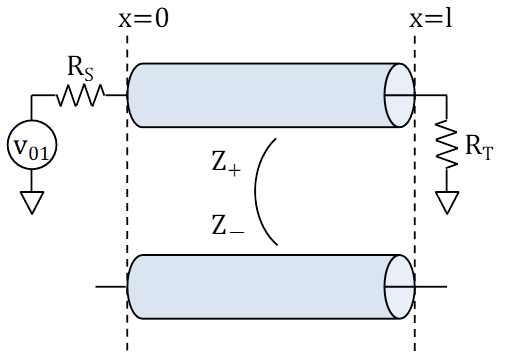
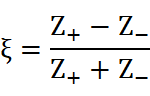Crosstalk noise onto a floating line
|
An active line-1 is terminated by resistances at both ends, and a passive line-2 is floating. The voltage on active line is the same in the case of a single line:
where
The voltage on passive line is proportional to that on active line:
where ξ is the crosstalk coefficient:
An example waveform for a rising step input v01(t) with rise time tr=τ/2:
|
We will calculate the crosstalk voltage onto a floating line.
***
We start from the general solutions in even and odd mode:
![]()
![]()
Transforming the variables of even and odd mode to those of each line:
![]()
we obtain the general solutions for each line:
![]()
![]()
![]()
![]()
where we have assumed that the propagation speeds are equal in even and odd mode.
![]()
For convenience, expressing (3),(4) in terms of Z0 and parameter ξ
![]()
,we have
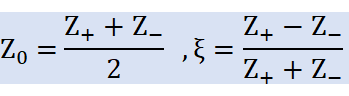
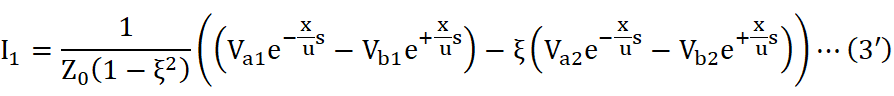
***
We are now ready to calculate the crosstalk noise onto a floating line.
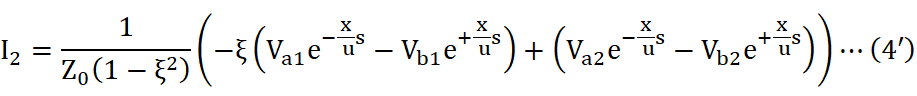
Boundary conditions are:
![]()
![]()
then Laplace transformed boundary conditions are
![]()
![]()
***
First, inserting the general solutions (1),(3') into the boundary conditions of line-1:
![]()
![]()
Eliminating the pair of Va2,Vb2:
![]()
![]()
After some manipulation we obtain:
![]()
![]()
![]()
where τ is the propagation time from x=0 to x=l, and ΓS,ΓT are reflection coefficients at source and termination end.
![]()
This result is the same in the case of a single line. (→Multiple reflection in a terminated line)
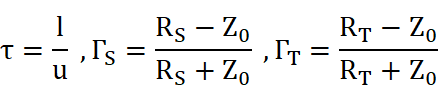
***
Next, inserting the general solutions (2),(4') into the boundary conditions of line-2:
![]()
![]()
Eliminating the pair of Vb1,Vb2 or Va1,Va2:
![]()
![]()
We find that the voltage on passive line-2 is proportional to that on active line-1.
![]()
This parameter ξ indicates the ratio of amplitude between passive and active line, so it is called crosstalk coefficient.