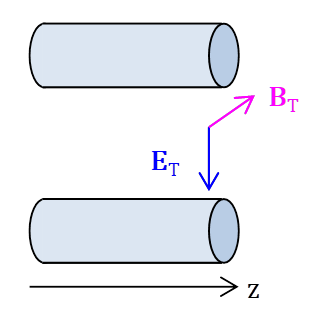
Distributed L and C model of a transmission line
|
Consider a uniform two-conductor transmission line.
Assuming that the electromagnetic wave propagates in a TEM mode, the voltage and current satisfies the equations:
where L and C are the inductance and capacitance per unit length along the transmission line. |
We will derive the equations of distributed L and C model.
***
We consider a uniform two-conductor transmission line and assume that the electromagnetic wave propagates in a TEM mode.
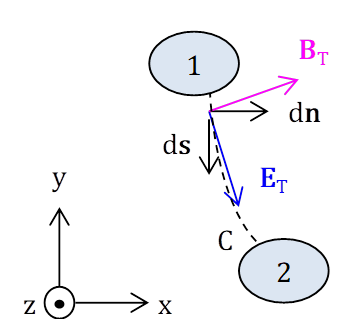
The voltage between conductor-1 and 2 is obtained by integrating ET・ds along any path C from conductor-1 to 2 :
![]()
![]()
![]()
![]()
![]()
![]()
![]()
Therefore the gradient of voltage v(z,t) is proportional to the time derivative of current i(z,t), and the proportional coefficient is the inductance between conductor-1 and 2:
![]()
***
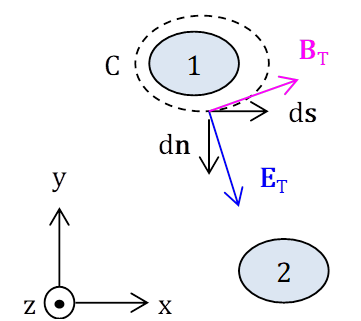
The current of conductor-1 is obtained by integrating BT・ds along any closed path C around conductor-1:
![]()
![]()
![]()
![]()
![]()
![]()
![]()
Therefore the gradient of current i(z,t) is proportional to the time derivative of voltage v(z,t), and the proportional coefficient is the capacitance between conductor-1 and 2:
![]()