TEM mode solution of Maxwell's equations
|
Maxwell's equations in a space among conductors of transmission line are:
The transverse electric and magnetic (TEM) mode solutions are:
where the suffix T indicates the transverse components:
The scalar potential φ satisfies the 2-D Laplace equation and the boundary condition:
|
We will derive the TEM mode solution in a transmission line.
***
In a space among conductors of transmission line, electric charges or currents do not exist. Then Maxwell's equations are:
![]()
![]()
![]()
![]()
Decomposing the fields into frequency components by Fourier transformation:
![]()
we obtain Maxwell's equations in the frequency domain:
![]()
![]()
![]()
![]()
Note that the last two equations automatically hold because of the first two equations.
For convenience, decomposing the fields into the transverse (suffix T) and longitudinal (suffix z) components:
![]()
![]()
, we obtain the four equations:
![]()
![]()
![]()
![]()
***
Assuming the transverse electric and magnetic (TEM) mode :
![]()
, the equations are simplified:
![]()
![]()
![]()
![]()
Eliminating BT between (2) and (4):
![]()
![]()
![]()
, we finally obtain the wave equation for ET.
Because of eq (1), ET can be expressed as a gradient of a scalar potential φ(x,y):
![]()
Substituting this into the wave equation:
![]()
, we obtain the TEM mode solution for ET.
![]()
Because the divergence of E is zero, the scalar potential φ(x,y) must satisfy the 2D-Laplace's equation:
![]()
![]()
Note that u is the propagation speed:
![]()
Substituting the solution ET into (2), we obtain BT :
![]()
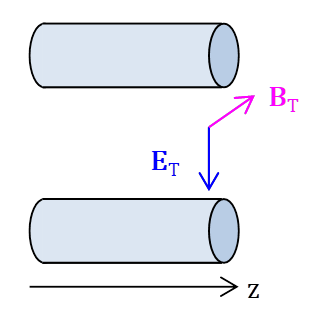
The directions of the electromagnetic fields and propagation direction z are depicted as follows: