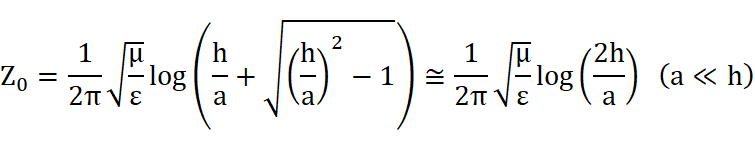Characteristic impedance of a wire over a ground plane
|
The characteristic impedance of a wire over a ground plane:
|
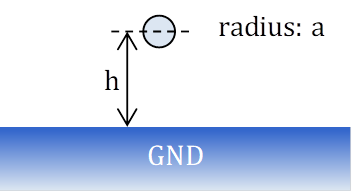
We will calculate the characteristic impedance, using an analytically solvable example: a wire over a ground plane.
***
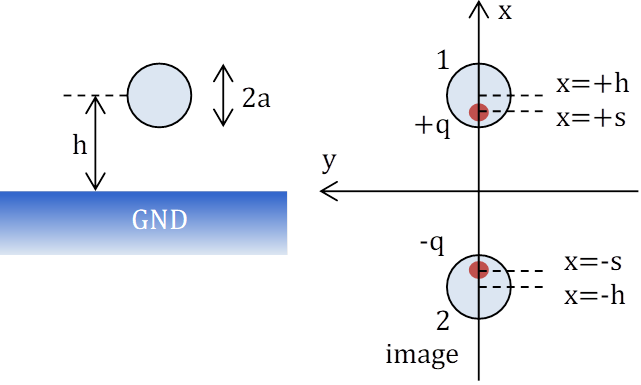
First, we will calculate the capacitance per unit length between the wire and plane. It is convenient to replace the wire and plane with a line-charge +q at (s,0) and its mirror image.
According to Gauss's law, the electric field E created by a line-charge q is
![]()
Therefore, the potential at distance r from the line is
![]()
Let the potential at the ground plane (x=0) be zero, the potential at a position (x,y) created by the line-charges +q and -q is
![]()
![]()
Then the equipotential line is a circle.
![]()
We determine the parameter s and K to fit the equipotential line in the outline of the wires.
![]()
![]()
These two solutions correspond to the potential of the wire 1 and 2 respectively.
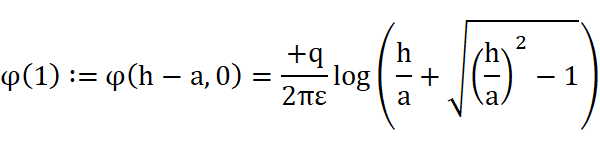
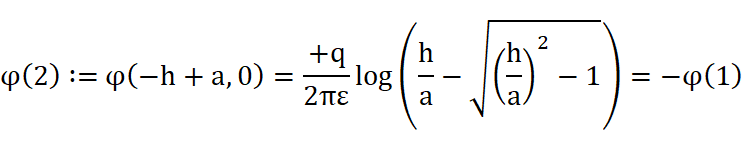
We obtain the capacitance between the wire and ground plane.
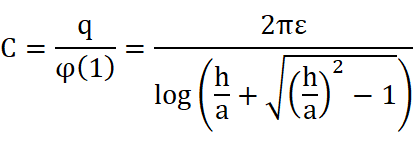
***
Next, we will calculate the inductance per unit length of the wire. It is convenient to replace the wire and plane with a line-current +I at (s,0) and its mirror image.
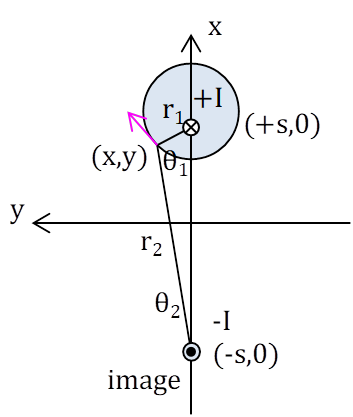
According to Ampere's law, the magnetic field H created by a line-current I is
![]()
Therefore, the total magnetic field at point (x,y) created by the two line-currents is
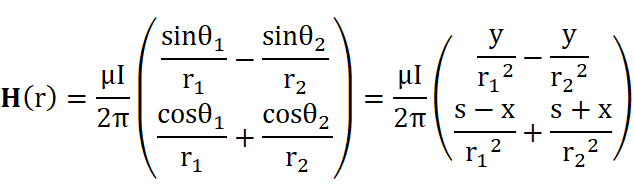
The magnetic field H must be perpendicular to the radius of the wire.
![]()
Then the line of magnetic force is a circle.
![]()
We determine the parameter s to fit the line of magnetic force in the outline of the wire.
![]()
The magnetic flux created by the line-current I, passing between the wires, is

Then the total magnetic flux created by the line-current +I and -I is
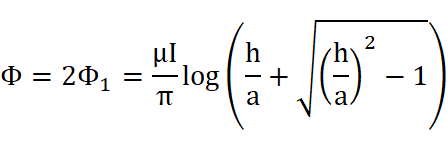
The magnetic flux between the wire and the ground plane is half of that between two wires. So we obtain the inductance of the wire over the ground plane.
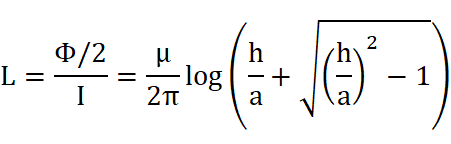
***
Using the capacitance and inductance above, we obtain the characteristic impedance.
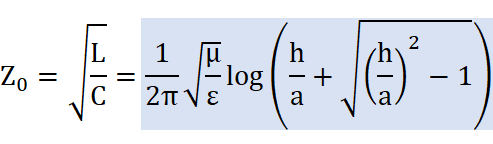
Similarly, the propagation speed is:
![]()
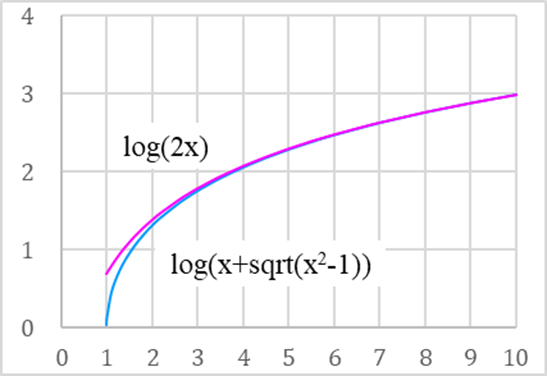
Note that the propagation speed does not depend on the form of conductors.
Assuming the radius a is much smaller than the height h, the form factor is simplified.
![]()
The permittivity and permeability of vacuum are
![]()
![]()
,and those of a material are
![]()
![]()
where εr is the relative permittivity of the material.
|
Material |
εr |
|
Glass-reinforced epoxy (FR-4) |
4.0-4.8 |
|
Polyethylene |
2.3 |
|
Air |
1.0 |
Then the characteristic impedance and the propagation speed are computed as
![]()
![]()